Содержание
- 2. Question 4. Find the limit of the sequence Solution. Our sequence can be written down as
- 3. Stolz-Cesaro Theorem Let an and bn be two sequences of real numbers. Assume that: is increasing
- 4. Thus, the Stolz-Cesaro Theorem tells us that To find the limit either apply the Stolz-Cesaro Theorem
- 5. apply the Stolz-Cesaro Theorem to or write it down as a product and then use the
- 6. Question 0: Answers to Questions from Light #1: Sequences and Limits Question 1: Question 4: Question
- 7. Calculus++ Also known as Hysterical Calculus
- 8. Question 1a. Find the following limit Solution. Use the Stolz-Cesaro theorem. In this case The sequence
- 9. The Stolz-Cesaro Theorem tells us that Hence
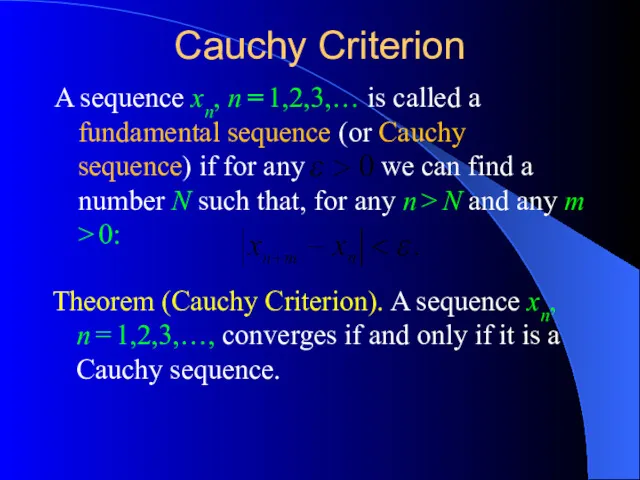
- 10. Cauchy Criterion A sequence xn, n = 1,2,3,… is called a fundamental sequence (or Cauchy sequence)
- 11. Definition (of non-fundamental sequences). A sequence xn, n = 1,2,3,… is not a Cauchy sequence if
- 12. Question 3. The sequence –1, +1, –1, +1,… is not a Cauchy sequence (and, hence, it
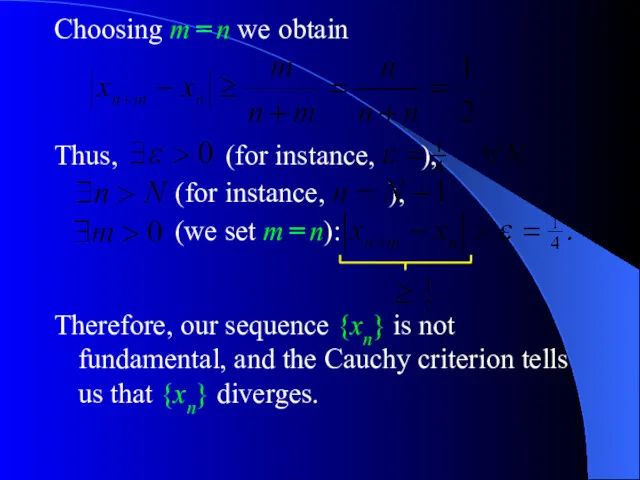
- 13. Question 4. Use the Cauchy criterion to show that the sequence diverges. Solution: According to the
- 14. Therefore, our sequence {xn} is not fundamental, and the Cauchy criterion tells us that {xn} diverges.
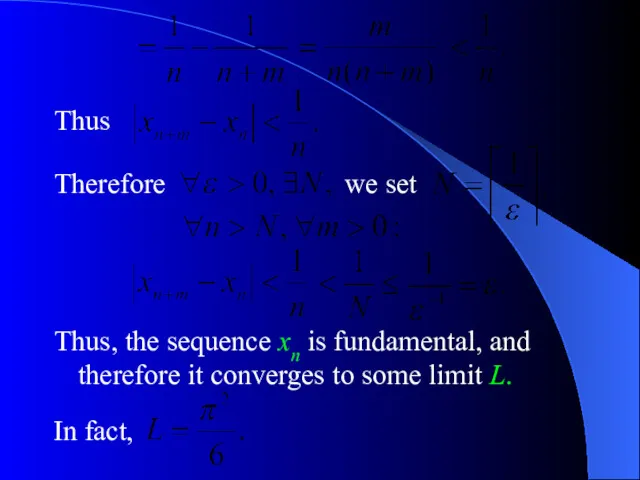
- 15. Question 5. Use the Cauchy criterion to show converges. Solution: It is sufficient to show that
- 16. Thus we set Therefore Thus, the sequence xn is fundamental, and therefore it converges to some
- 17. Picture of the Week
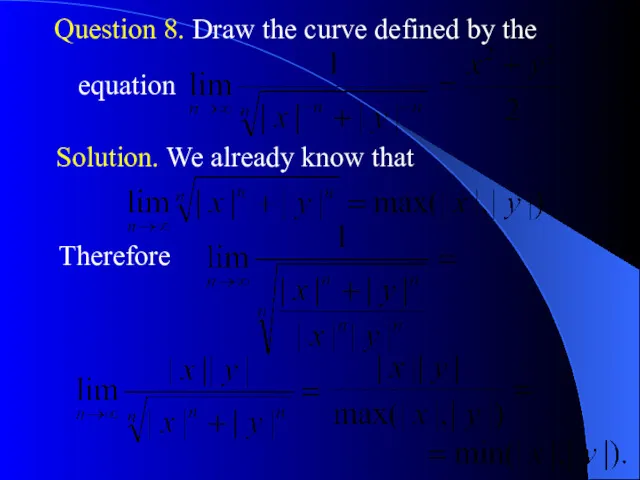
- 18. Question 8. Draw the curve defined by the Solution. We already know that Therefore equation
- 19. Thus, we have to draw the curve defined by the equation
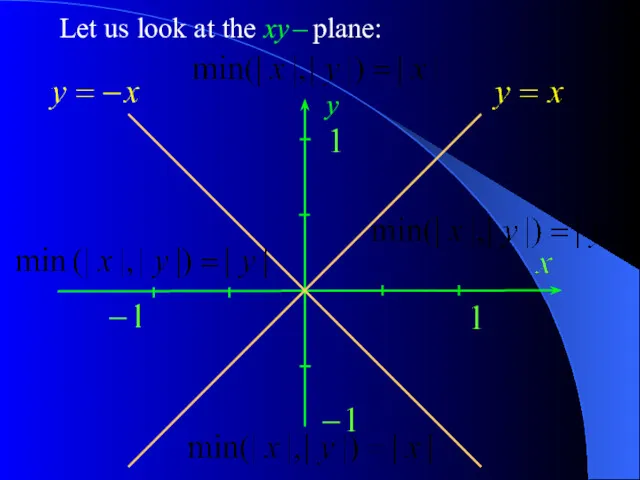
- 20. Let us look at the xy – plane: y
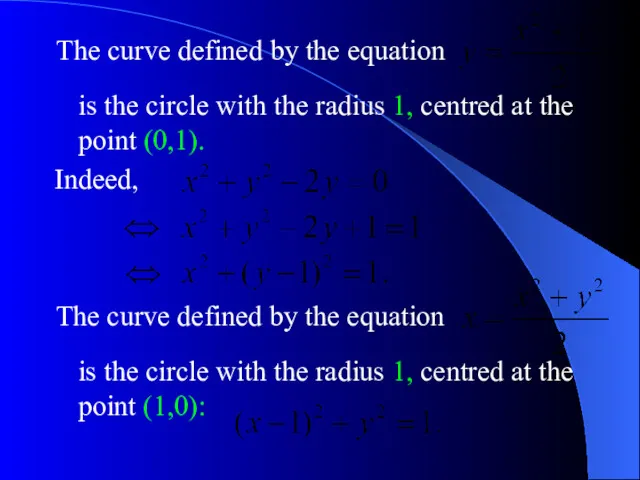
- 21. The curve defined by the equation is the circle with the radius 1, centred at the
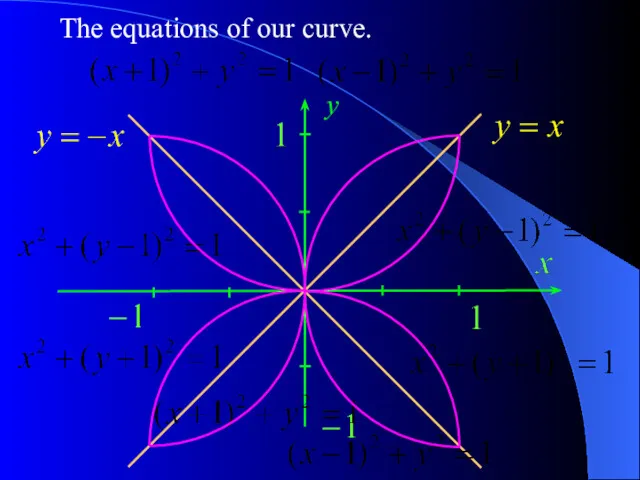
- 22. The equations of our curve. y
- 23. The picture of the week. y
- 25. Скачать презентацию















 Распределительное свойство умножения
Распределительное свойство умножения Метод крутого восхождения
Метод крутого восхождения Сфера. Шар. Части сферы и шара
Сфера. Шар. Части сферы и шара Задачи на готовых чертежах
Задачи на готовых чертежах Формулы для вычисления площадей различных треугольников
Формулы для вычисления площадей различных треугольников Множества. Отношения между множествами
Множества. Отношения между множествами Сложение чисел с разными знаками
Сложение чисел с разными знаками Особенности урока математики в начальной школе в соответствии с ФГОС ΙΙ поколения.
Особенности урока математики в начальной школе в соответствии с ФГОС ΙΙ поколения. Угол между прямыми в пространстве
Угол между прямыми в пространстве Математические модели в экономике
Математические модели в экономике Қарапайым математикалық түсініктер. Уақытты бағдарлау
Қарапайым математикалық түсініктер. Уақытты бағдарлау Задачи на движение в разных направлениях (схемы)
Задачи на движение в разных направлениях (схемы) урок математики в 1 классе
урок математики в 1 классе Системы линейных уравнений. Ранг матрицы
Системы линейных уравнений. Ранг матрицы Магія чисел. Перевірка домашнього завдання
Магія чисел. Перевірка домашнього завдання Угол. Прямой и развернутый угол. 5 класс
Угол. Прямой и развернутый угол. 5 класс Трапеция. 8 класс
Трапеция. 8 класс Интерактивная игра Цвет и форма
Интерактивная игра Цвет и форма Тренажер по математике №2. Сложение и вычитание в пределах 10
Тренажер по математике №2. Сложение и вычитание в пределах 10 Метод координат в решении задач С2
Метод координат в решении задач С2 Симметрия относительно плоскости
Симметрия относительно плоскости Решение уравнений
Решение уравнений Квадратные уравнения. Решение уравнений
Квадратные уравнения. Решение уравнений Как писать цифры
Как писать цифры Поворот точки вокруг начала координат
Поворот точки вокруг начала координат Использование на уроках математики программы Geogebra и платформы Гуглкласс
Использование на уроках математики программы Geogebra и платформы Гуглкласс Устный счет для 1 класса
Устный счет для 1 класса Дидактическая электронная игра
Дидактическая электронная игра