Quantum Mechanics 2: Schroedinger equation. Atomic wave functions. Atomic orbitals. Quantum numbers презентация
Содержание
- 2. Wave Functions In quantum mechanics a particle cannot be described using trajectory. Rather, it is best
- 3. Wave functions are often complex functions (have both real and imaginary part) and have coordinates as
- 4. We postulate that there exist a wave function that describes distribution of electron is space. Because
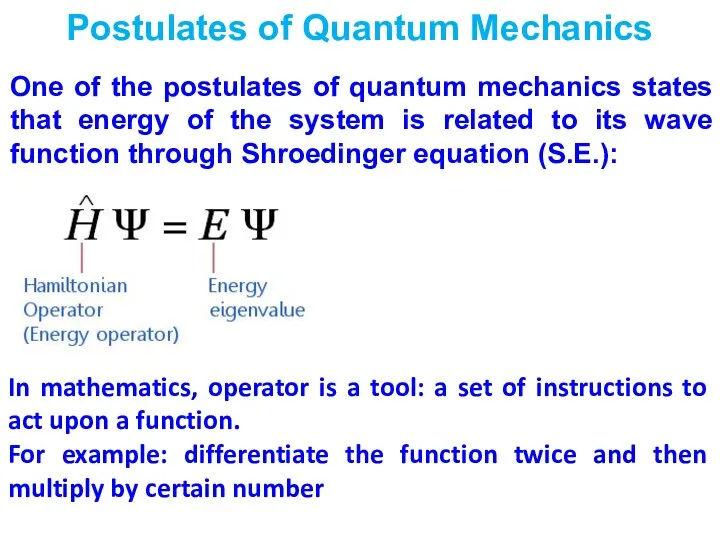
- 5. Postulates of Quantum Mechanics One of the postulates of quantum mechanics states that energy of the
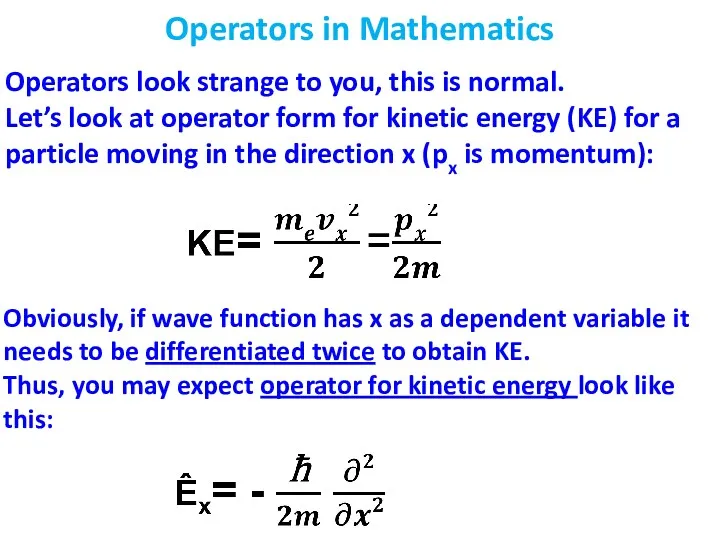
- 6. Operators in Mathematics Operators look strange to you, this is normal. Let’s look at operator form
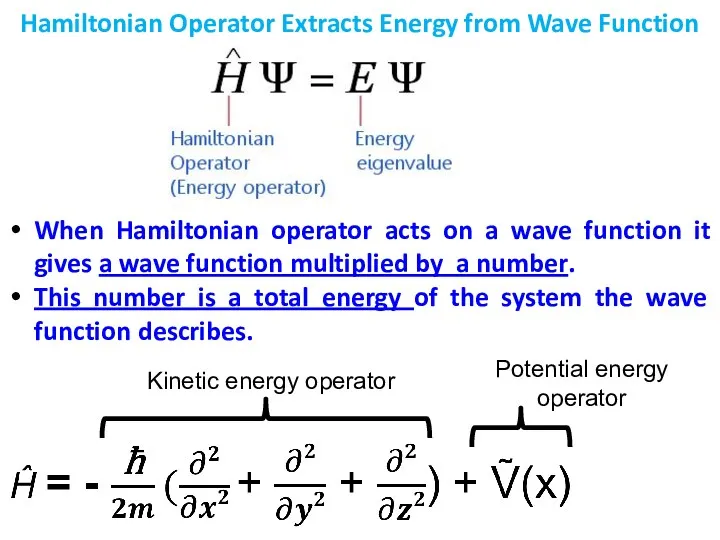
- 7. Hamiltonian Operator Extracts Energy from Wave Function When Hamiltonian operator acts on a wave function it
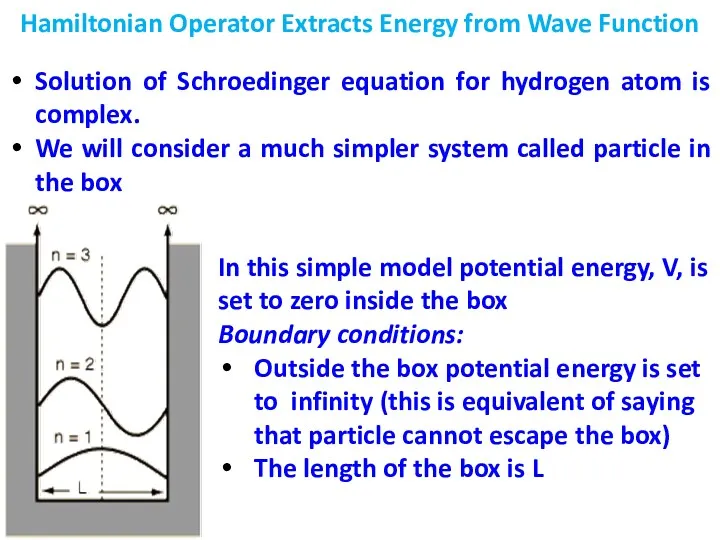
- 8. Hamiltonian Operator Extracts Energy from Wave Function Solution of Schroedinger equation for hydrogen atom is complex.
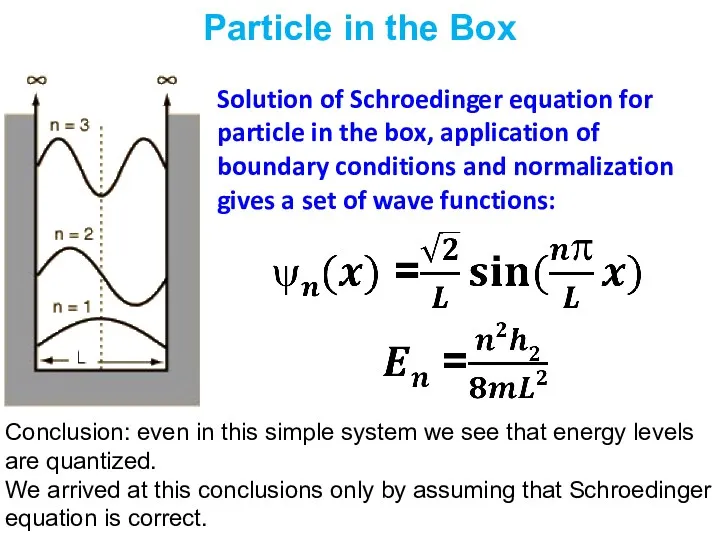
- 9. Particle in the Box Solution of Schroedinger equation for particle in the box, application of boundary
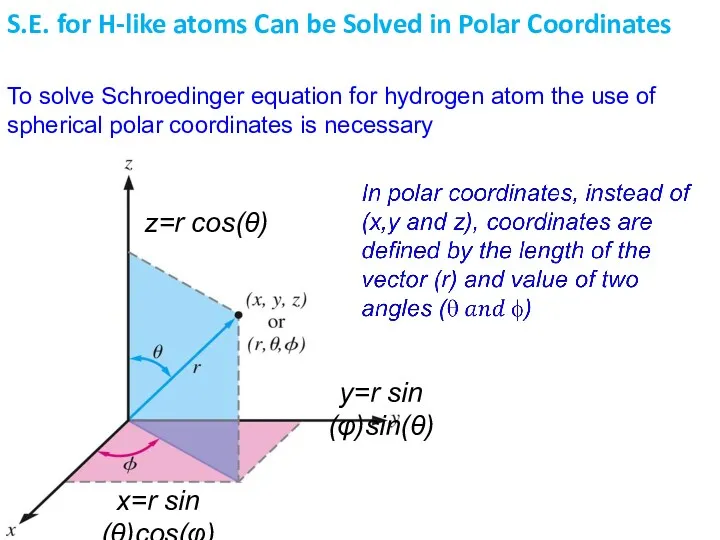
- 10. To solve Schroedinger equation for hydrogen atom the use of spherical polar coordinates is necessary S.E.
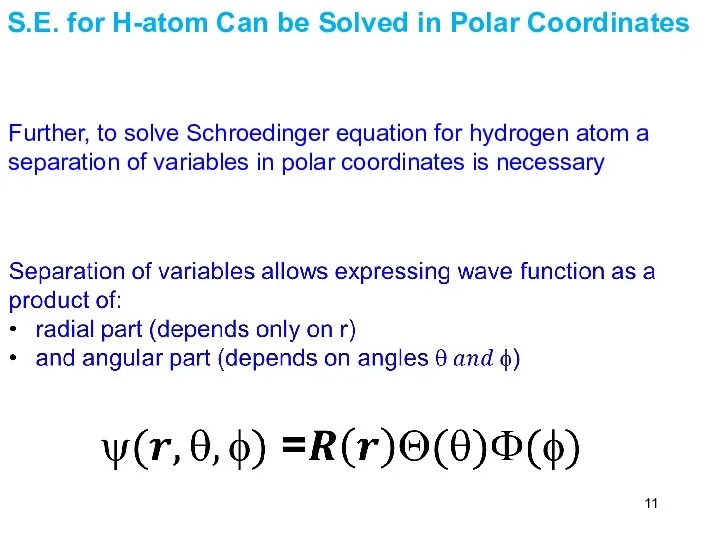
- 11. Further, to solve Schroedinger equation for hydrogen atom a separation of variables in polar coordinates is
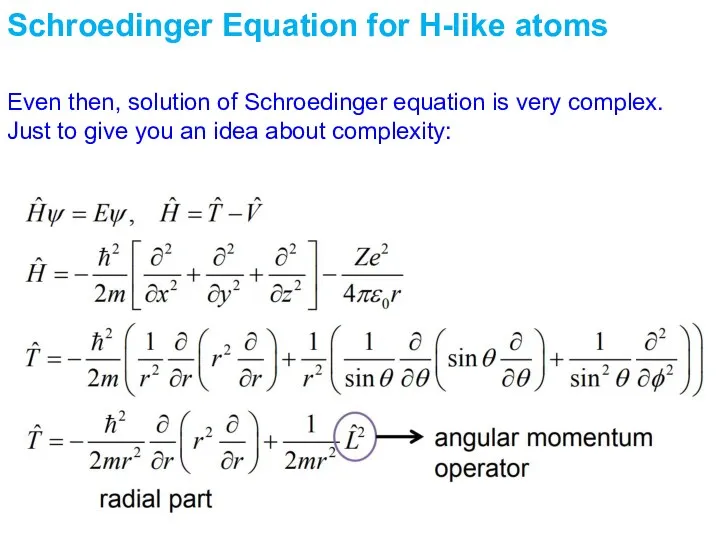
- 12. Even then, solution of Schroedinger equation is very complex. Just to give you an idea about
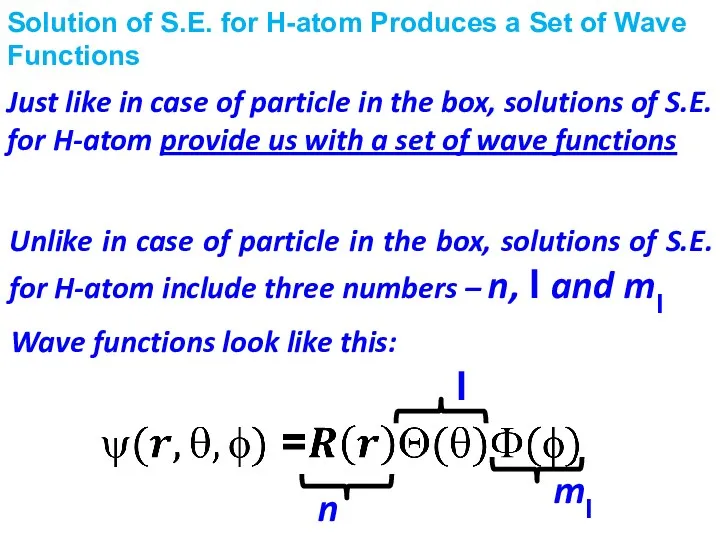
- 13. Solution of S.E. for H-atom Produces a Set of Wave Functions Unlike in case of particle
- 14. Example of a Wave Function Corresponding wave function looks like this: Mathematically, the simplest solution of
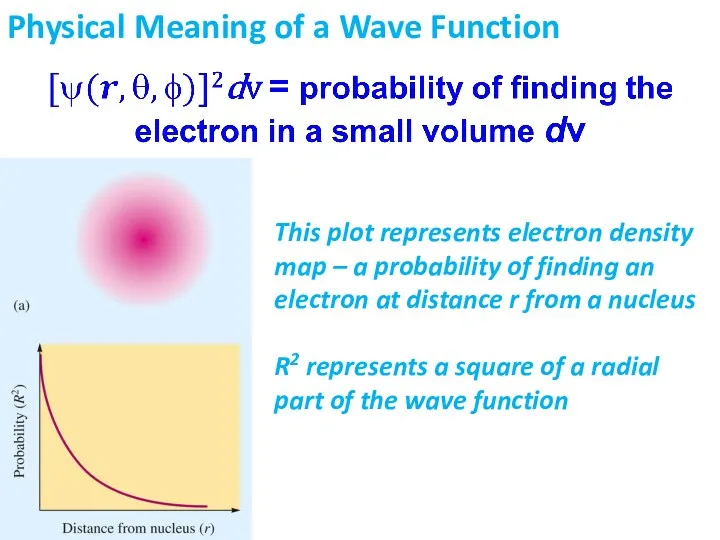
- 15. Physical Meaning of a Wave Function This plot represents electron density map – a probability of
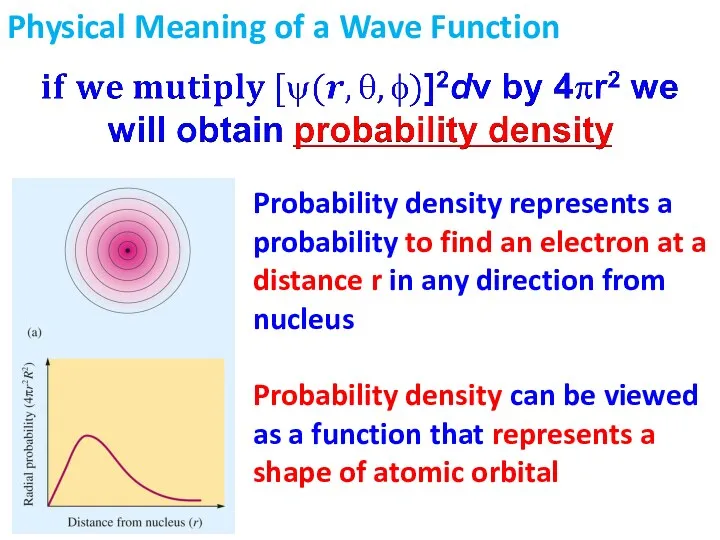
- 16. Physical Meaning of a Wave Function Probability density represents a probability to find an electron at
- 17. Solutions of Schroedinger Equation of Single-Electron Atom Only real parts of wave functions are shown
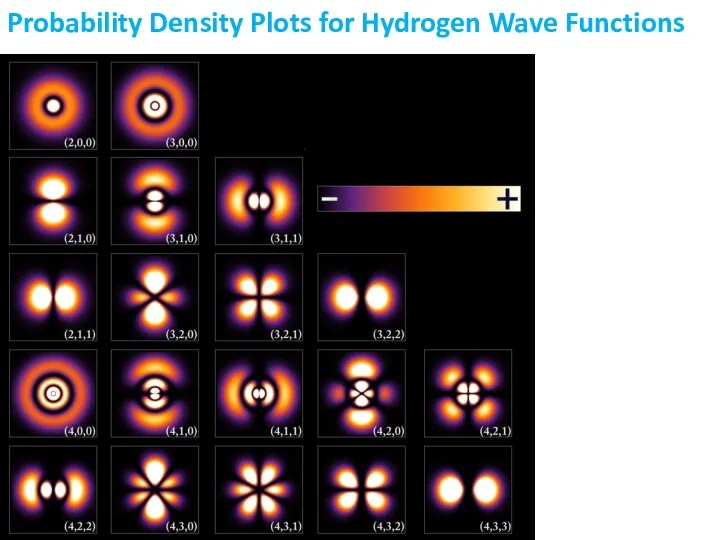
- 18. Probability Density Plots for Hydrogen Wave Functions
- 19. Each orbital has a unique probability distribution which we can schematically depict as a shape of
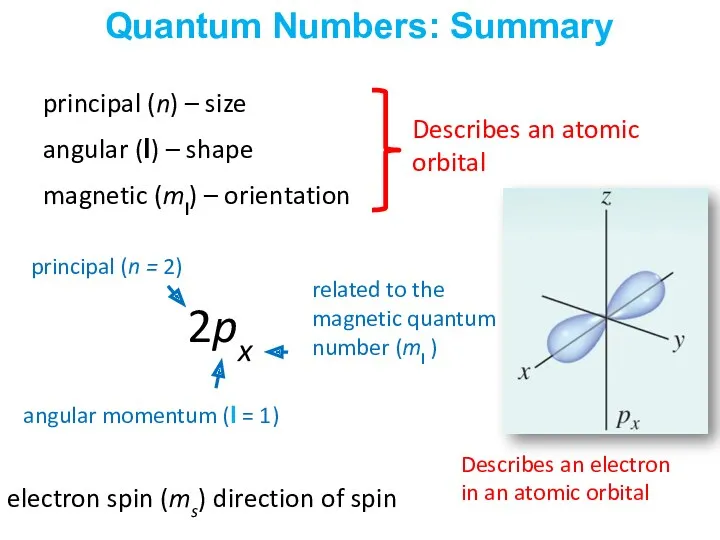
- 20. Quantum numbers are required to describe the distribution of electron density in an atom. There are
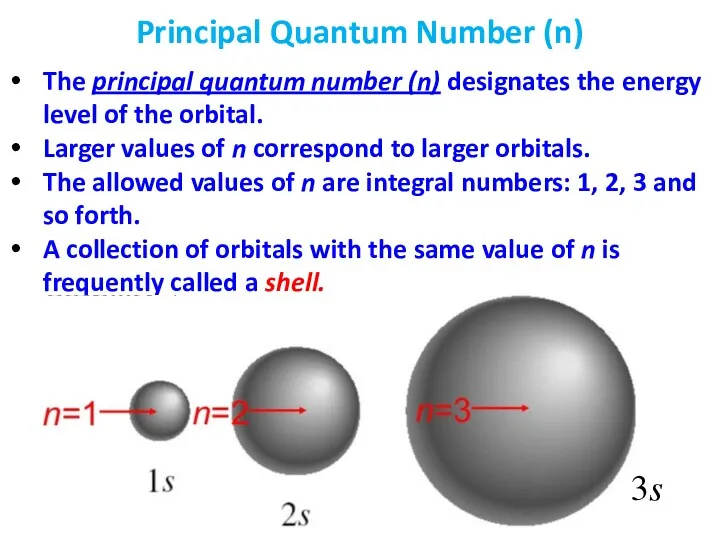
- 21. The principal quantum number (n) designates the energy level of the orbital. Larger values of n
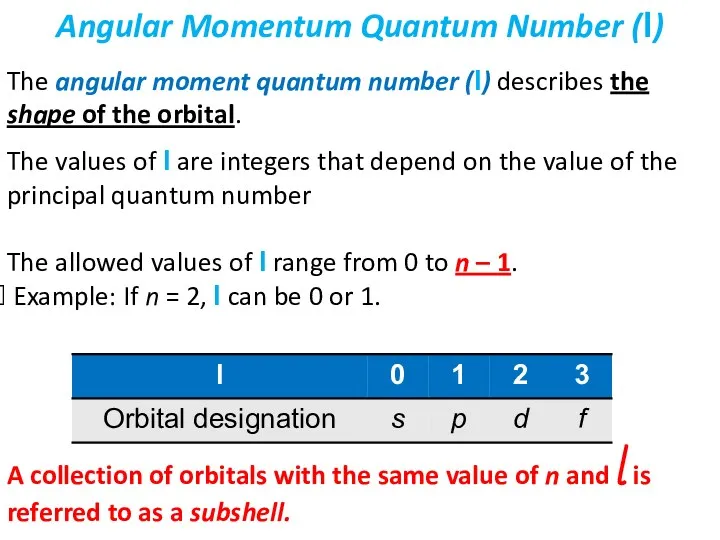
- 22. The angular moment quantum number (l) describes the shape of the orbital. The values of l
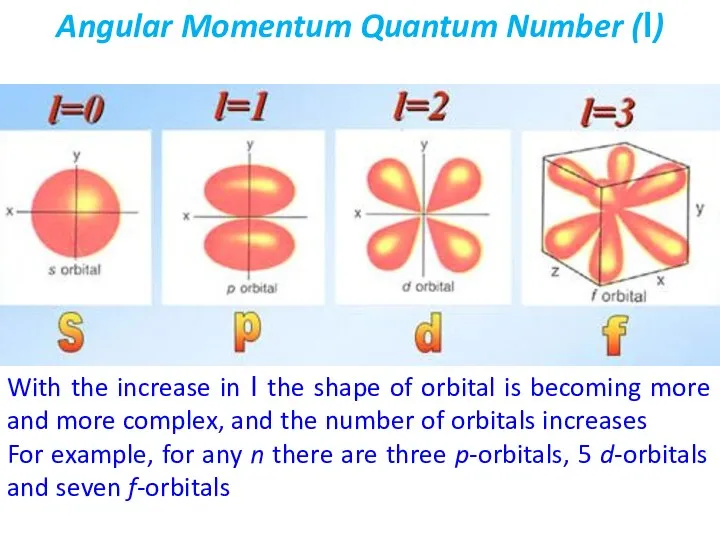
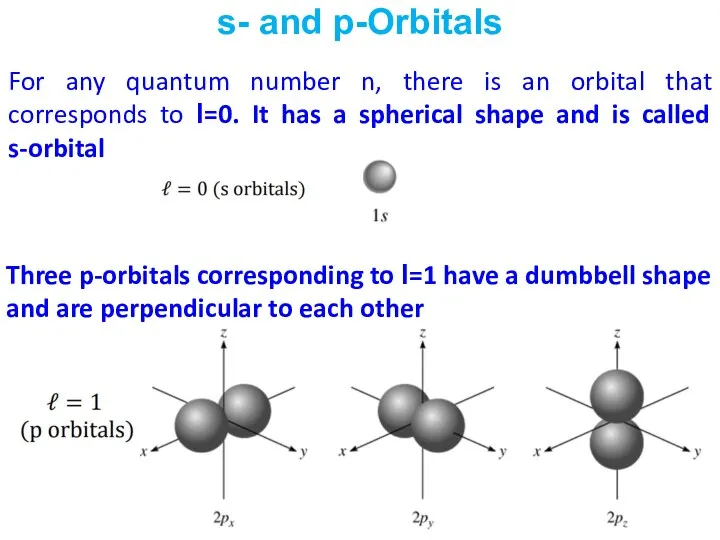
- 23. Angular Momentum Quantum Number (l) With the increase in l the shape of orbital is becoming
- 24. Three p-orbitals corresponding to l=1 have a dumbbell shape and are perpendicular to each other For
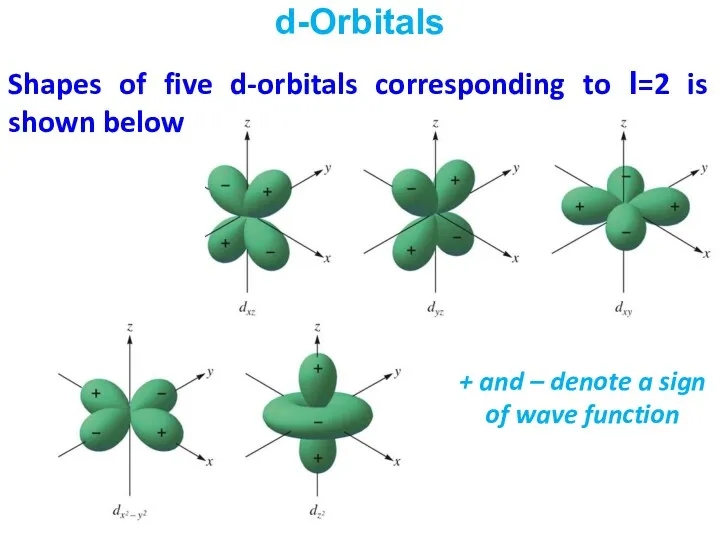
- 25. Shapes of five d-orbitals corresponding to l=2 is shown below d-Orbitals + and – denote a
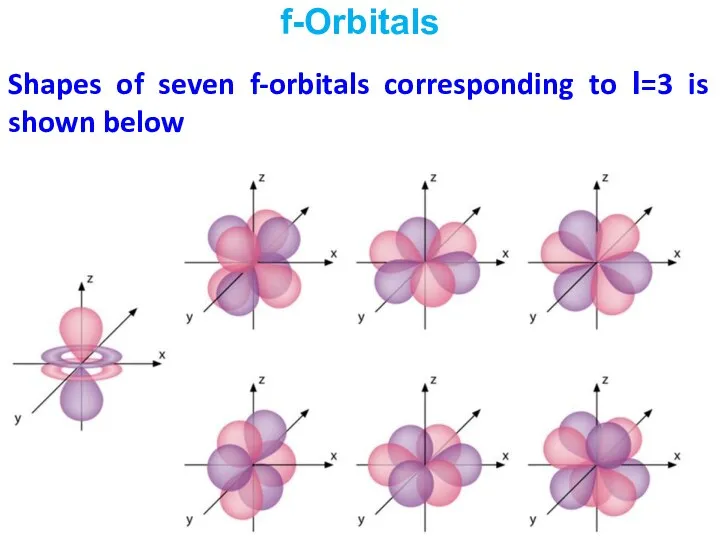
- 26. Shapes of seven f-orbitals corresponding to l=3 is shown below f-Orbitals
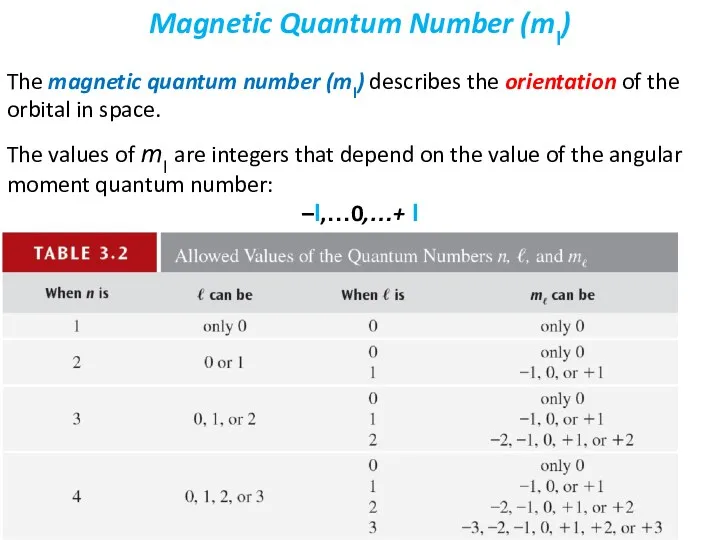
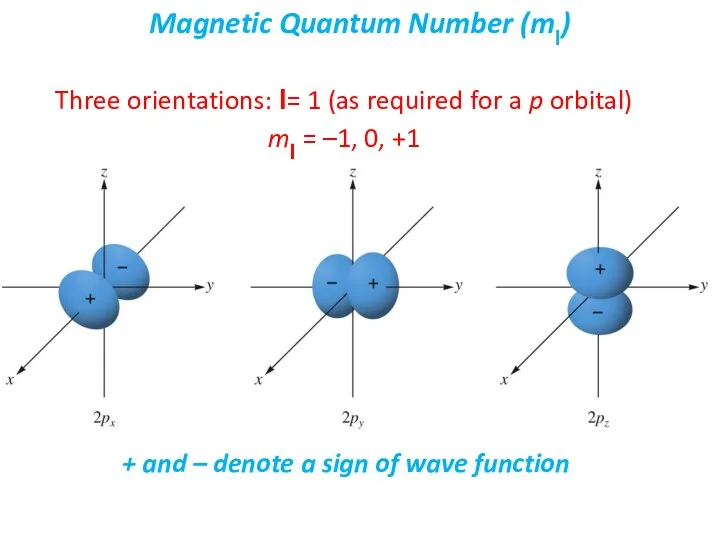
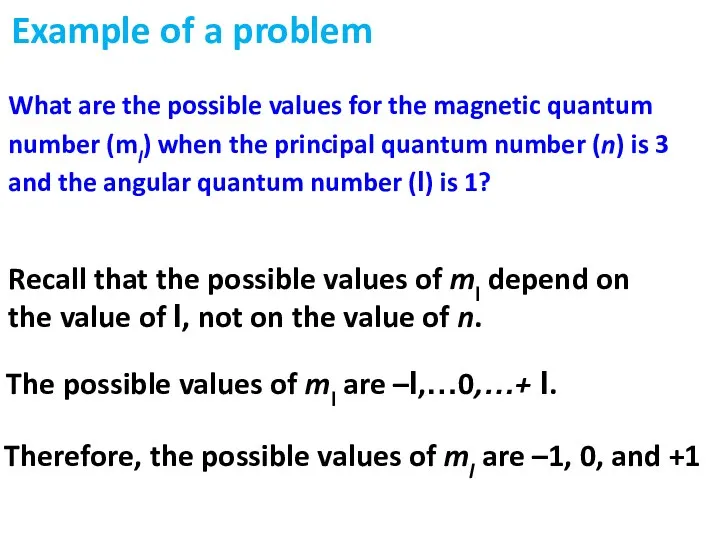
- 27. The magnetic quantum number (ml) describes the orientation of the orbital in space. The values of
- 28. Three orientations: l= 1 (as required for a p orbital) ml = –1, 0, +1 Magnetic
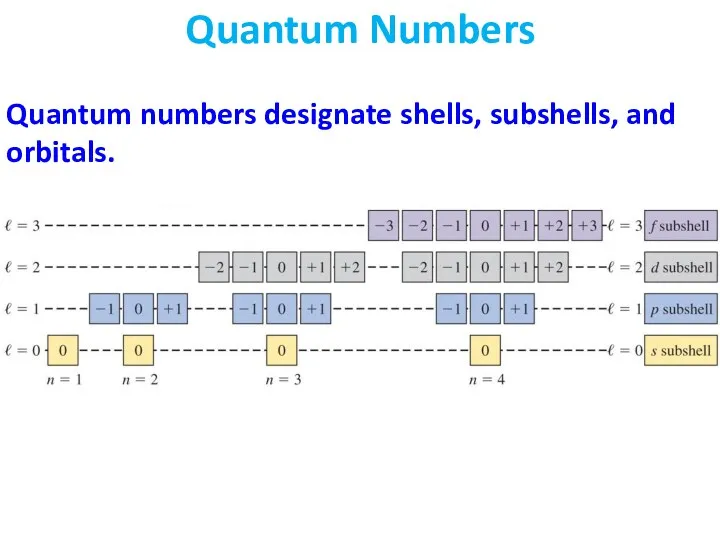
- 29. Quantum numbers designate shells, subshells, and orbitals. Quantum Numbers
- 30. Recall that the possible values of ml depend on the value of l, not on the
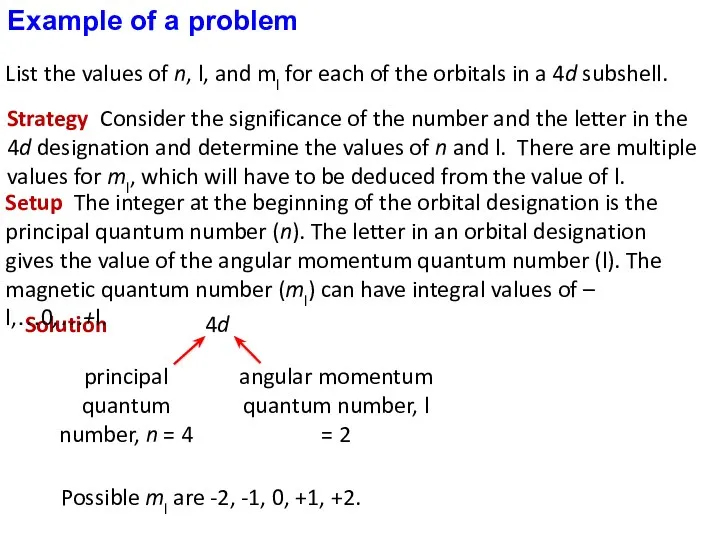
- 31. Strategy Consider the significance of the number and the letter in the 4d designation and determine
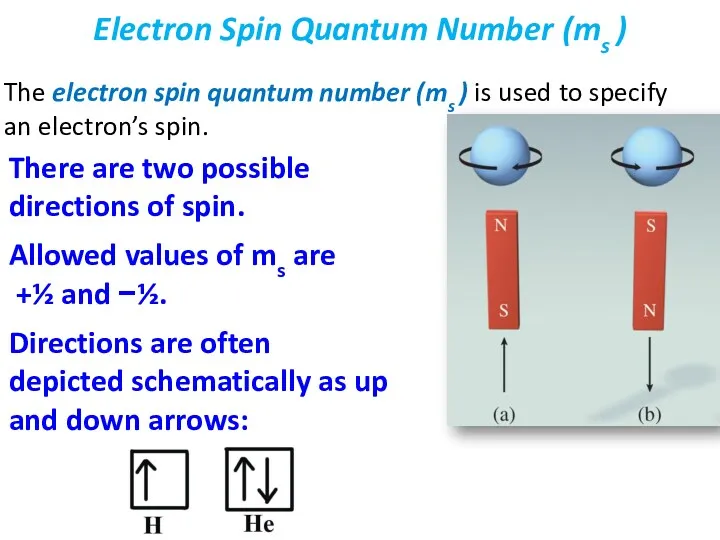
- 32. The electron spin quantum number (ms ) is used to specify an electron’s spin. Electron Spin
- 33. A beam of atoms is split by a magnetic field. Statistically, half of the electrons spin
- 34. principal (n) – size angular (l) – shape magnetic (ml) – orientation electron spin (ms) direction
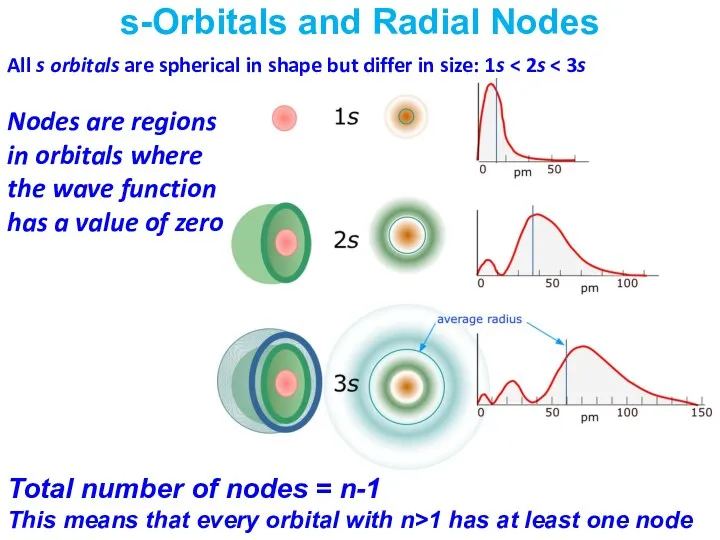
- 35. s-Orbitals and Radial Nodes Nodes are regions in orbitals where the wave function has a value
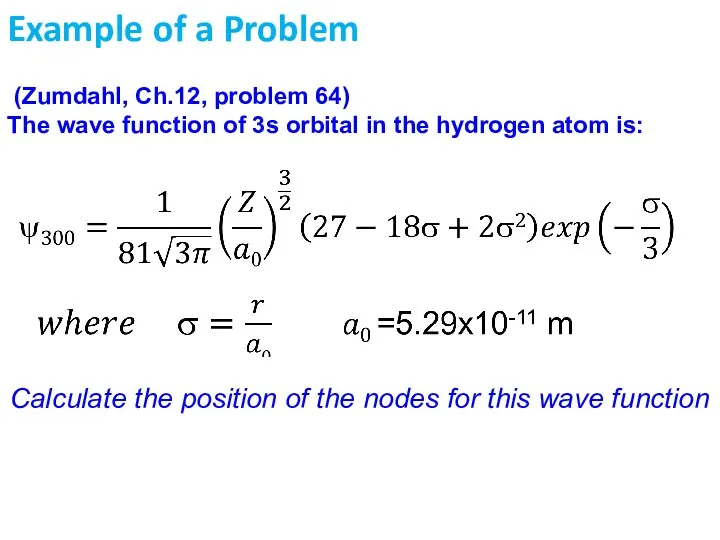
- 36. Example of a Problem (Zumdahl, Ch.12, problem 64) The wave function of 3s orbital in the
- 37. There may be 2 types of nodes in an orbital: Radial (spherical surface) Angular (plane or
- 38. p orbitals have one angular node – a plane in every point of which there is
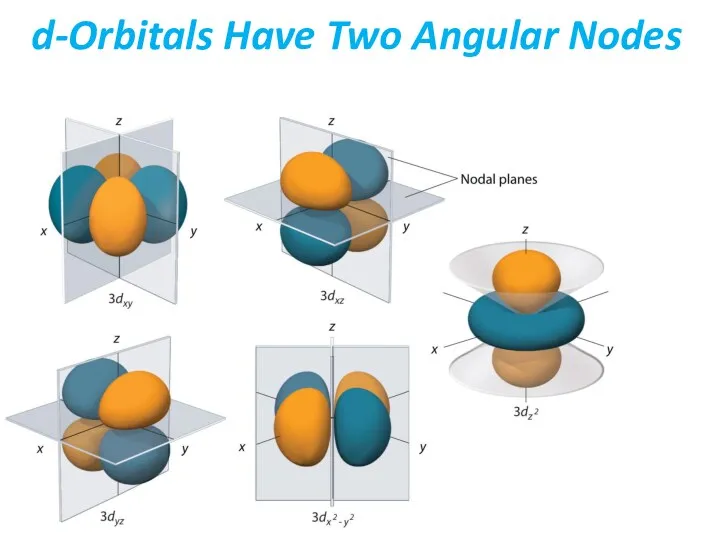
- 39. d-Orbitals Have Two Angular Nodes
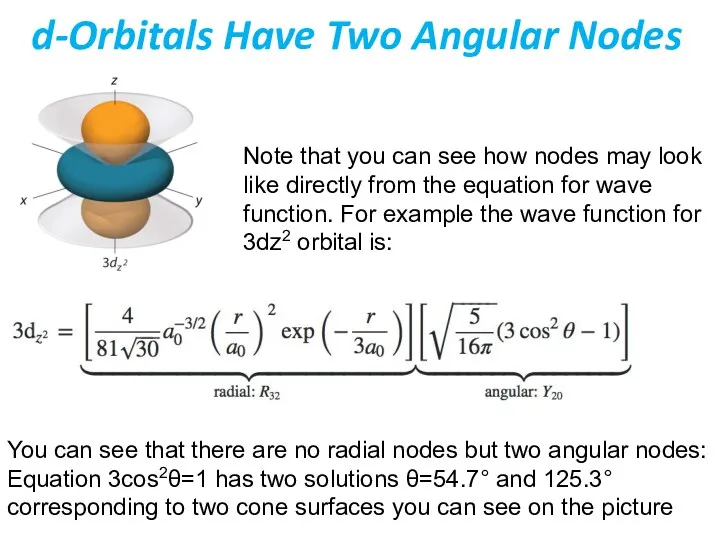
- 40. d-Orbitals Have Two Angular Nodes Note that you can see how nodes may look like directly
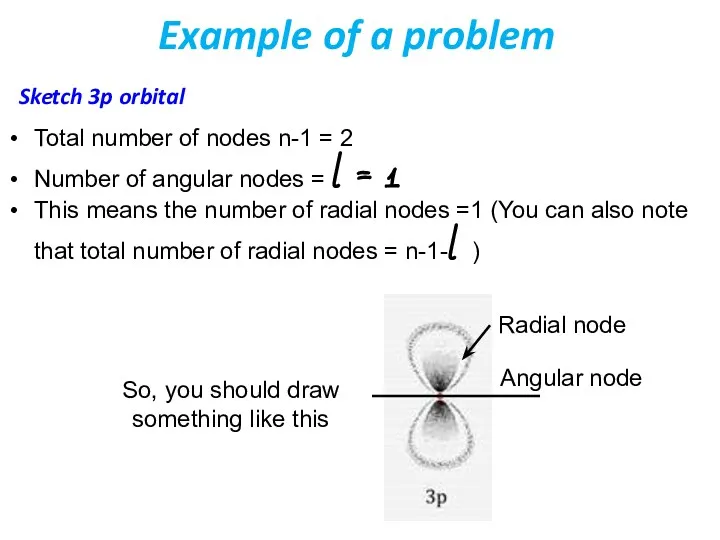
- 41. Example of a problem Sketch 3p orbital Total number of nodes n-1 = 2 Number of
- 42. S.E. for Polyelectronic Atoms Cannot be Solved in Analytical Form In polyelectronic atoms electrons influence each
- 43. Are you ready for Monday?
- 46. Скачать презентацию











 Волновая оптика
Волновая оптика Системы управления автомобилем
Системы управления автомобилем Увеличение мощности автомобильного двигателя
Увеличение мощности автомобильного двигателя Равномерное движение по окружности
Равномерное движение по окружности Резерфорд тәжірибесі. Атомның планетарлық моделі
Резерфорд тәжірибесі. Атомның планетарлық моделі Насыщенный пар
Насыщенный пар 濽á ÒÓÒúßÔ¿ ºá¬¡ âÒ¬á
濽á ÒÓÒúßÔ¿ ºá¬¡ âÒ¬á Газораспределительный механизм
Газораспределительный механизм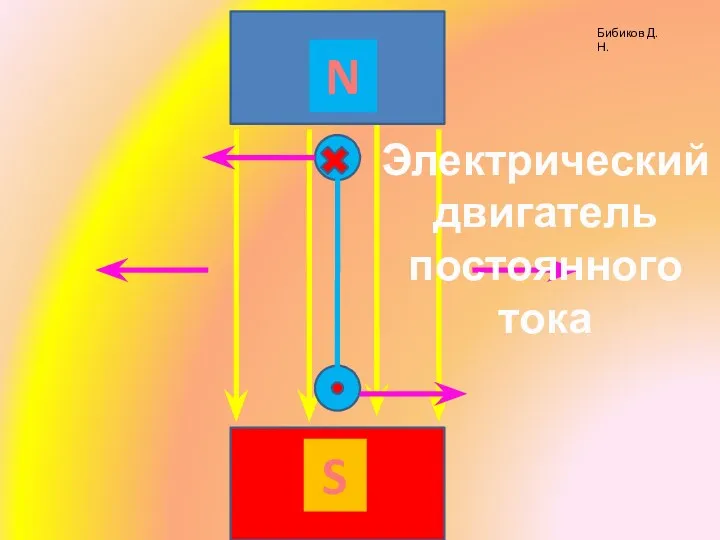 Электрические машины постоянного тока
Электрические машины постоянного тока Фізичні й хімічні явища у природі
Фізичні й хімічні явища у природі Урок повторения и обобщения 7 класс по теме: Давление
Урок повторения и обобщения 7 класс по теме: Давление Испарение и конденсация
Испарение и конденсация Общее устройство тракторов
Общее устройство тракторов Дифракция құбылысы. Френел және Фраунгофер жуықтаулары. Амплитудалық және фазалық дифракциялық торлар
Дифракция құбылысы. Френел және Фраунгофер жуықтаулары. Амплитудалық және фазалық дифракциялық торлар Элементтердің периодтық жүйесі және оның физикалық түсіндірілуі. Паули принципі. Хунд ережесі
Элементтердің периодтық жүйесі және оның физикалық түсіндірілуі. Паули принципі. Хунд ережесі Прочность, совместимость и радиационная стойкость реакторных материалов. Тема 3
Прочность, совместимость и радиационная стойкость реакторных материалов. Тема 3 Организация проектной деятельности учащихся
Организация проектной деятельности учащихся Электродинамика. Электростатика. Закон сохранения электрического заряда. Закон Кулона
Электродинамика. Электростатика. Закон сохранения электрического заряда. Закон Кулона Оценка последствий взрыва
Оценка последствий взрыва Повышение качества обработки колец подшипников
Повышение качества обработки колец подшипников Закон Ома для участка электрической цепи
Закон Ома для участка электрической цепи Investigation, of the use of planetary-circular gears in machine. Tool drives
Investigation, of the use of planetary-circular gears in machine. Tool drives Валы и оси
Валы и оси Организация технического обслуживания и ремонта автомобиля Шевроле Нива
Организация технического обслуживания и ремонта автомобиля Шевроле Нива Магнитное поле в вакууме
Магнитное поле в вакууме Машины постоянного тока. Устройство, материалы и принцип действия. Карточка 16
Машины постоянного тока. Устройство, материалы и принцип действия. Карточка 16 Механические передачи. Ременные передачи
Механические передачи. Ременные передачи Моделирование электрофизических свойств gaas методом монте-карло
Моделирование электрофизических свойств gaas методом монте-карло