Содержание
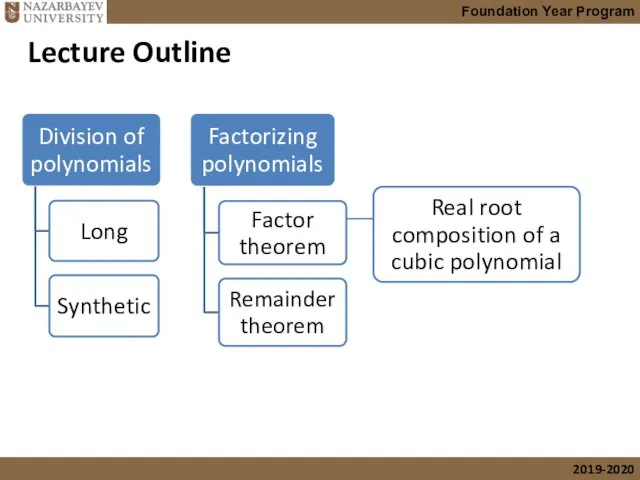
- 2. Lecture Outline
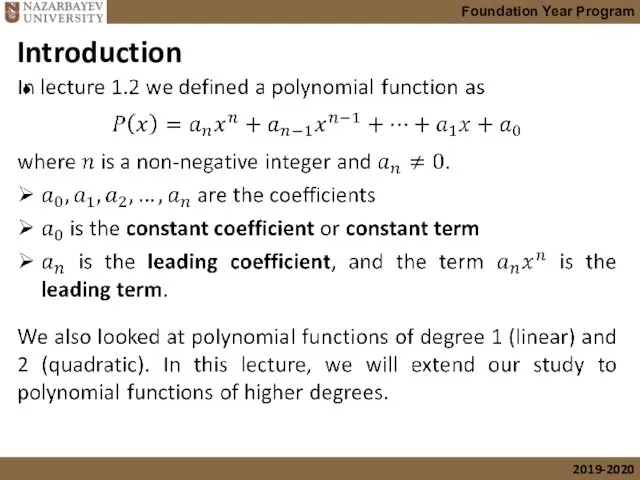
- 3. Introduction
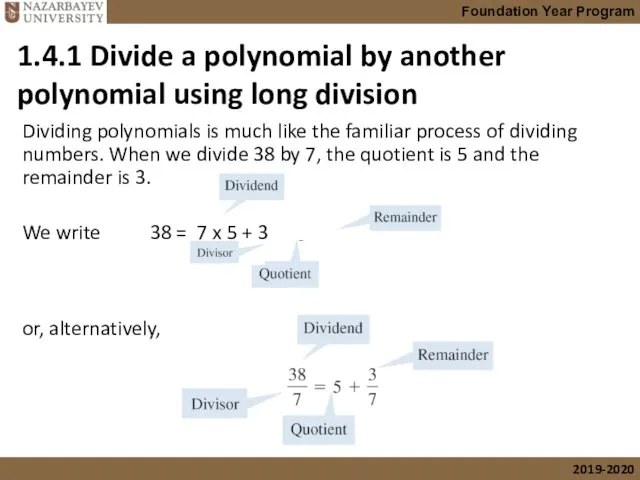
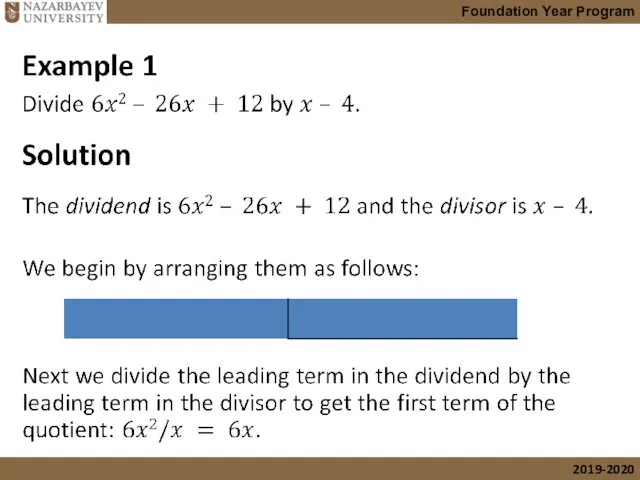
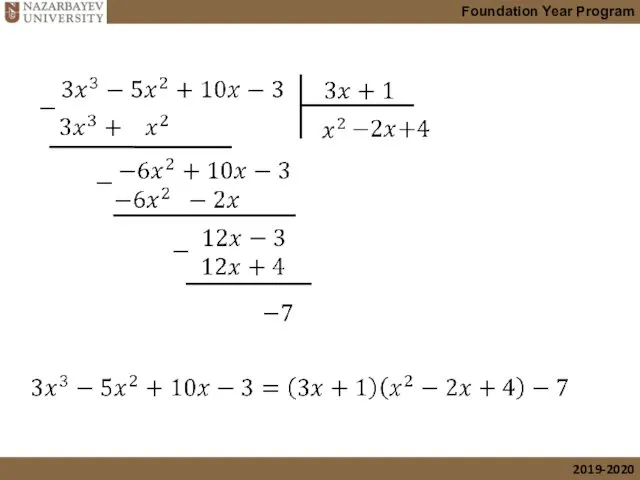
- 4. 1.4.1 Divide a polynomial by another polynomial using long division Dividing polynomials is much like the
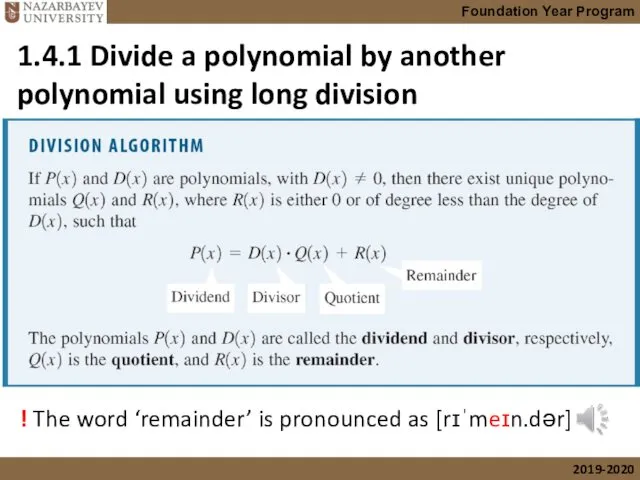
- 5. 1.4.1 Divide a polynomial by another polynomial using long division ! The word ‘remainder’ is pronounced
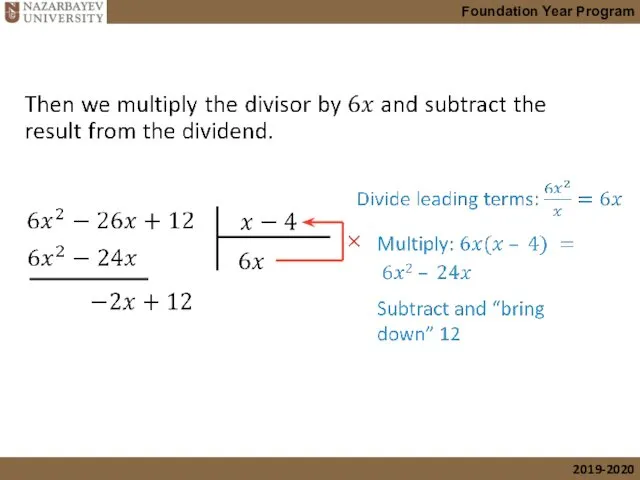
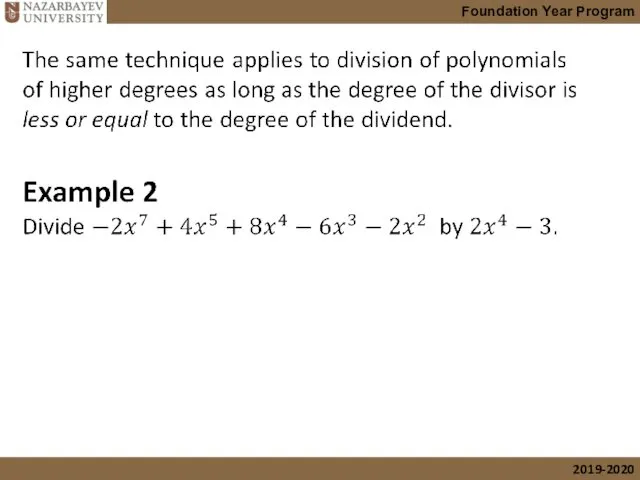
- 7. cont’d
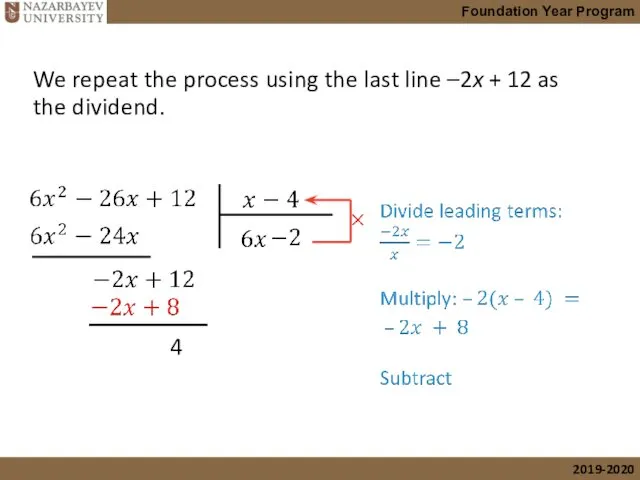
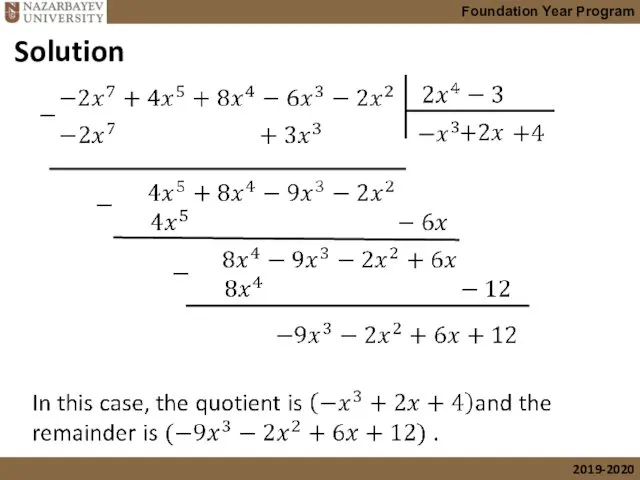
- 8. We repeat the process using the last line –2x + 12 as the dividend. 4
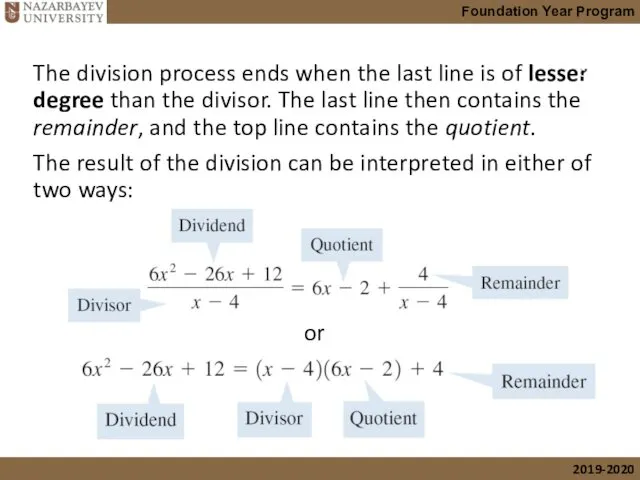
- 9. The division process ends when the last line is of lesser degree than the divisor. The
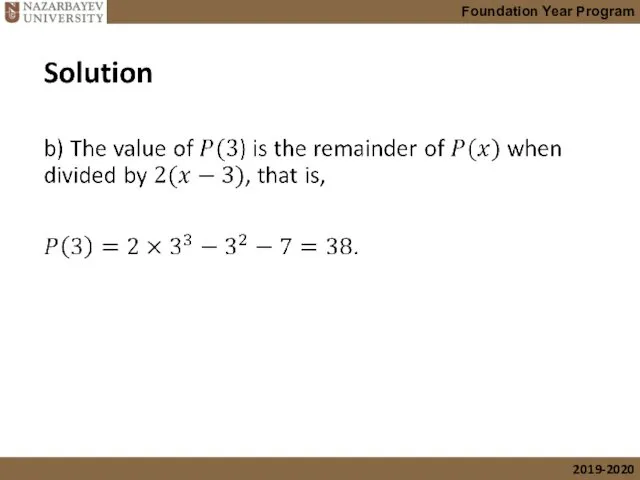
- 13. Solution
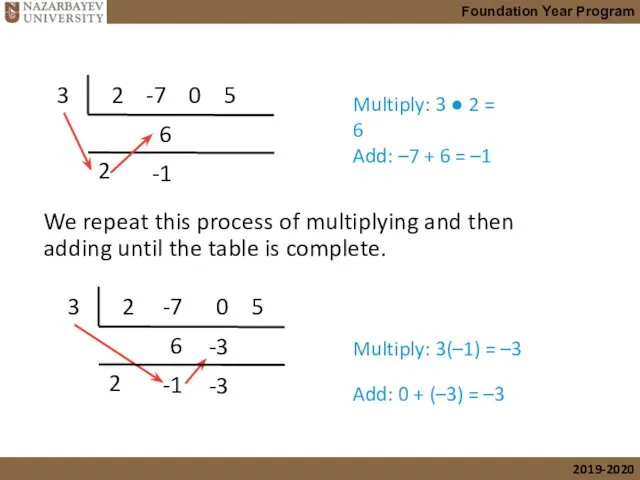
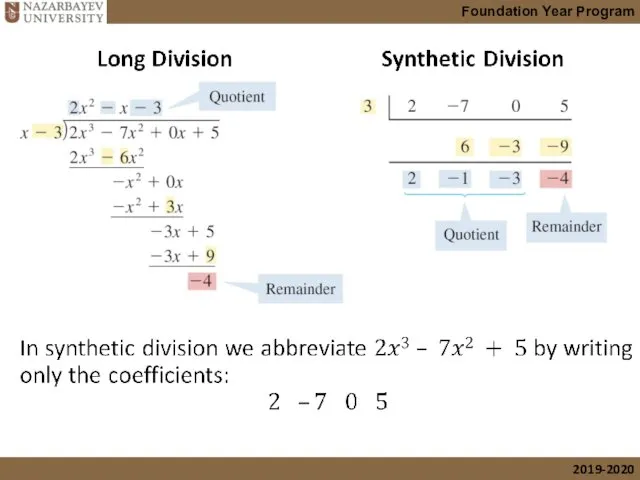
- 14. 1.4.2 Divide a polynomial by a linear polynomial using synthetic division
- 15. We will bring down the 2, multiply 3 ● 2 = 6, and write the result
- 16. cont’d Multiply: 3 ● 2 = 6 Add: –7 + 6 = –1 We repeat this
- 17. cont’d cont’d Multiply: 3(–3) = –9 Add: 5 + (–9) = –4 2 -7 0 5
- 19. cont’d
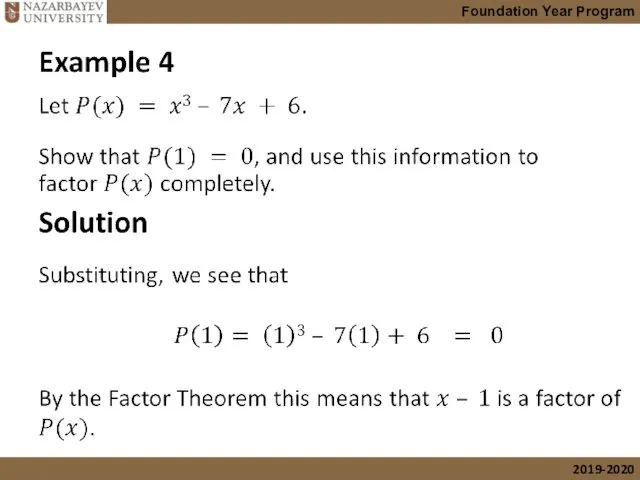
- 20. 1.4.3 Apply the factor theorem to factorize polynomials The next theorem says that zeros of polynomials
- 22. cont’d
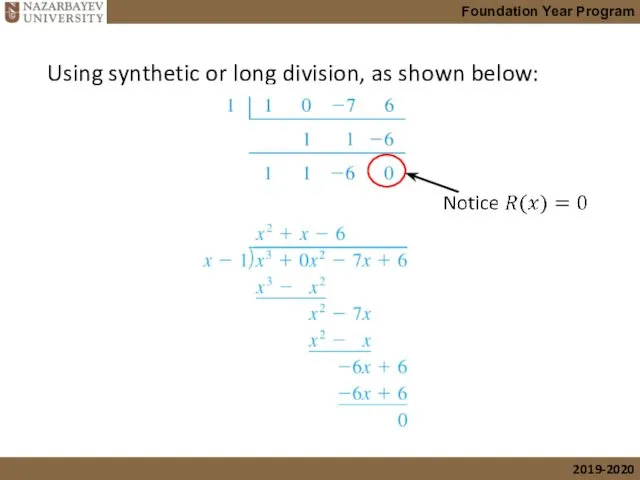
- 23. cont’d Using synthetic or long division, as shown below: cont’d
- 24. cont’d Given polynomial Using synthetic or long division Factor quadratic x2 + x – 6
- 25. cont’d
- 26. cont’d cont’d
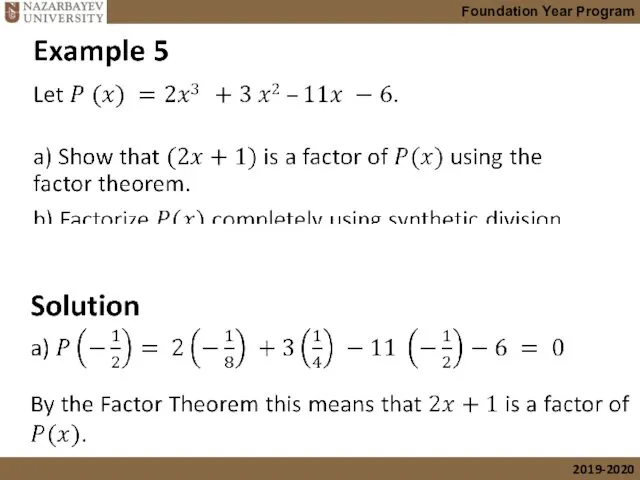
- 27. Solution
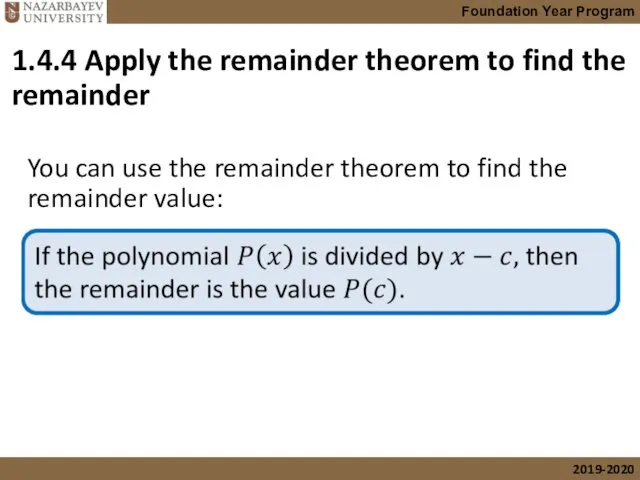
- 28. cont’d You can use the remainder theorem to find the remainder value: 1.4.4 Apply the remainder
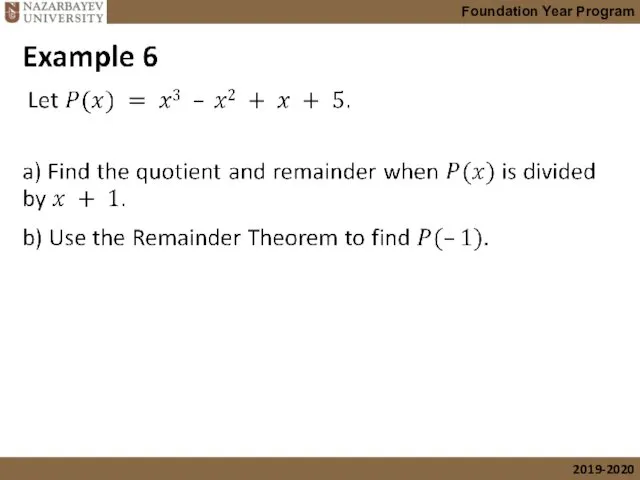
- 30. cont’d
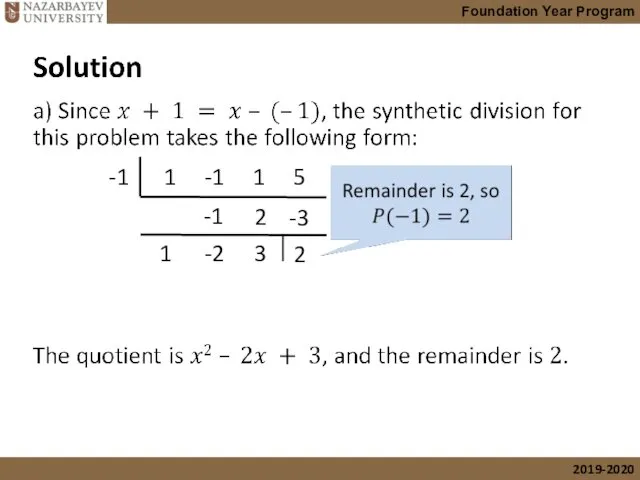
- 31. cont’d 1 -1 1 5 -1 1 -1 -2 2 3 -3 2
- 32. cont’d cont’d
- 33. cont’d Solution
- 34. cont’d
- 35. cont’d
- 36. cont’d Based on the previous exercise, we can state the following: Remainder Theorem – version 2
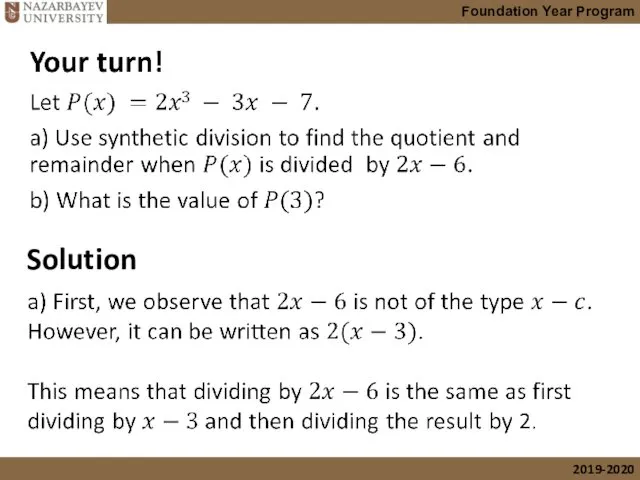
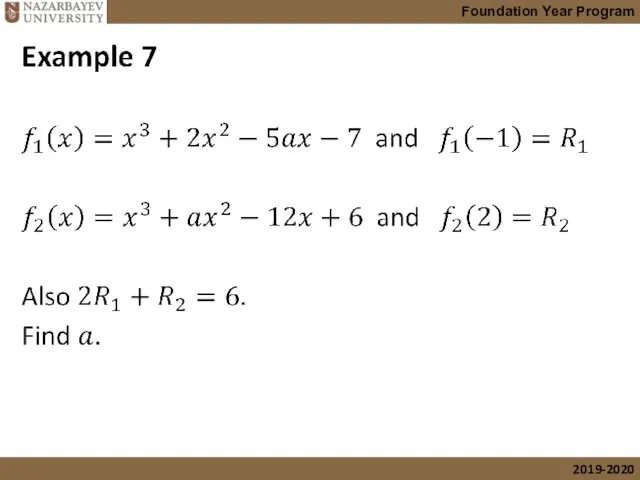
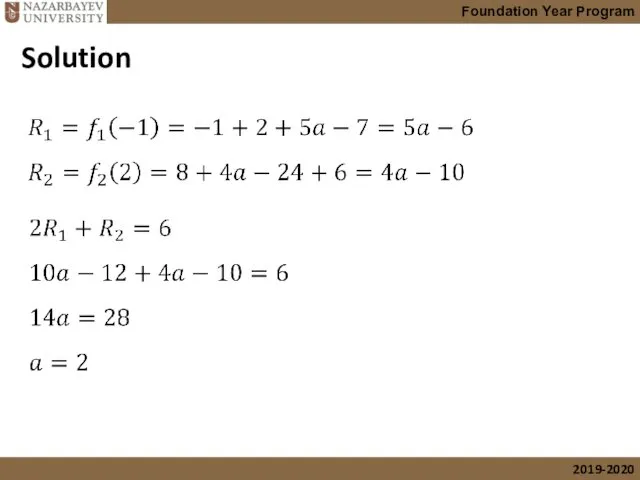
- 38. Solution
- 39. Your turn!
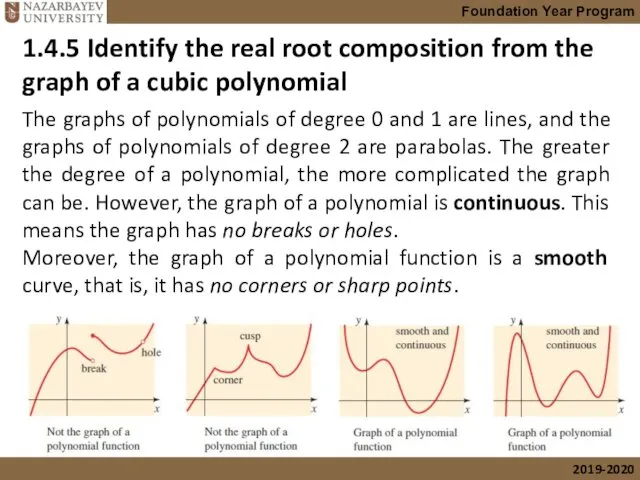
- 40. 1.4.5 Identify the real root composition from the graph of a cubic polynomial The graphs of
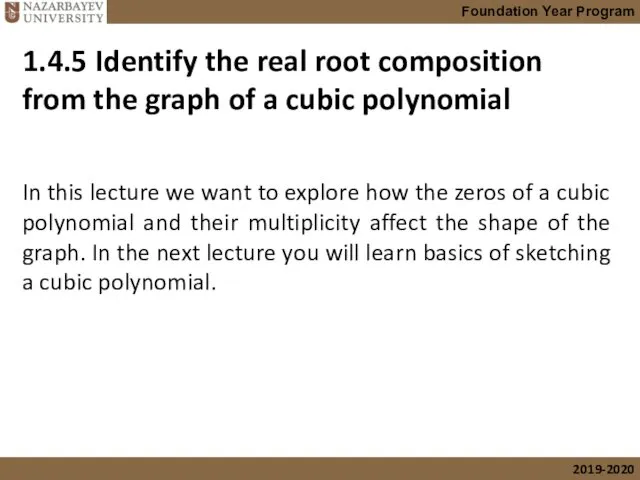
- 41. 1.4.5 Identify the real root composition from the graph of a cubic polynomial In this lecture
- 42. Case A: p(x) has a triple zero k>0 k
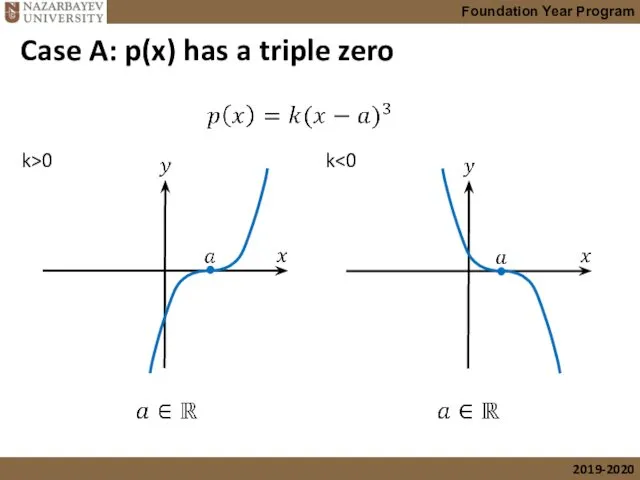
- 43. Case B: p(x) has a double zero k>0 k
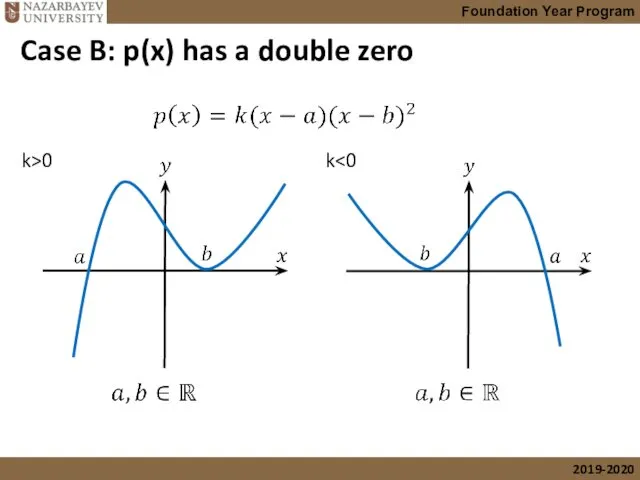
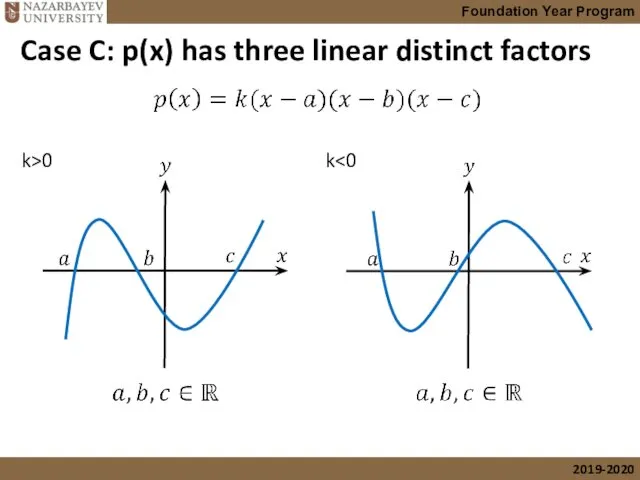
- 44. Case C: p(x) has three linear distinct factors k>0 k
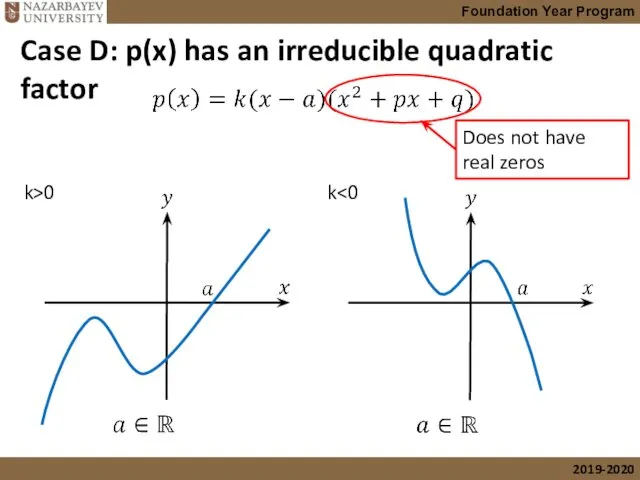
- 45. Case D: p(x) has an irreducible quadratic factor k>0 k Does not have real zeros
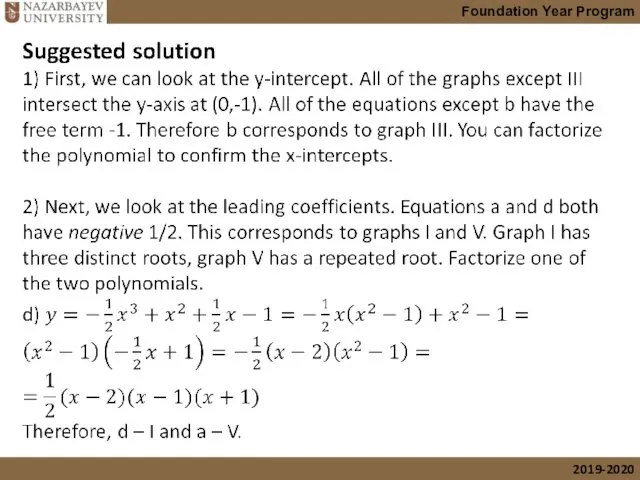
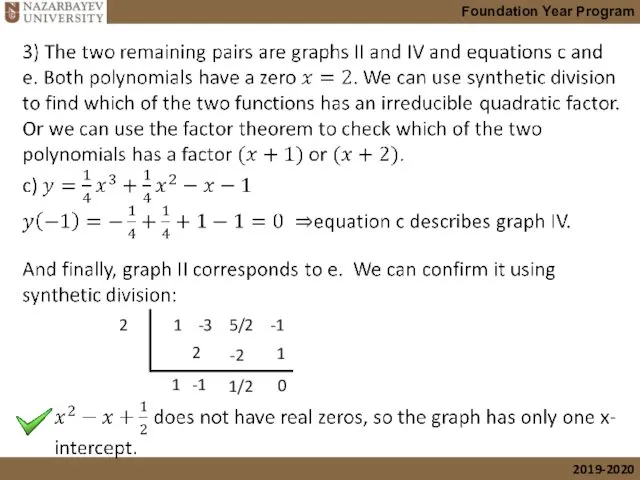
- 46. Your turn! Match the cubic function with one of the graphs shown. x y x y
- 48. 1 -3 5/2 -1 2 1 2 -1 -2 1/2 1 0
- 50. Learning outcomes 1.4.1 Divide a polynomial by another polynomial using long division 1.4.2 Divide a polynomial
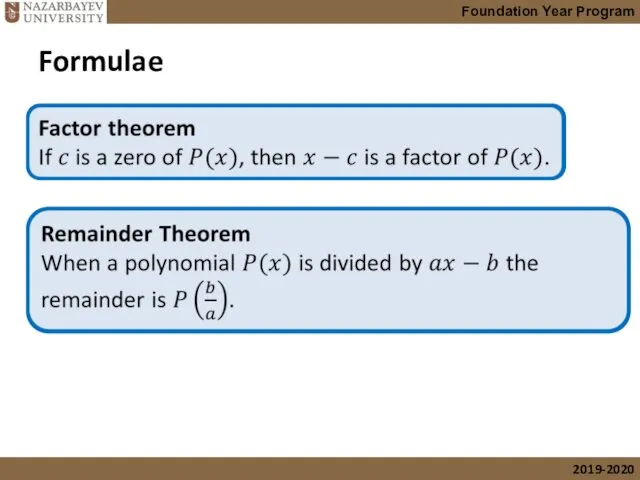
- 51. Formulae
- 53. Скачать презентацию
















 Австрийская республика
Австрийская республика Воинские звания действующие в РККА до 1943 года
Воинские звания действующие в РККА до 1943 года Урок Доброты
Урок Доброты Патентные базы данных компании Questel
Патентные базы данных компании Questel Транспорт в моем городе. Фрагмент урока географии.
Транспорт в моем городе. Фрагмент урока географии. Гестагенсодержащие контрацептивы
Гестагенсодержащие контрацептивы Онлайн-курс по продвижению бизнеса в Google
Онлайн-курс по продвижению бизнеса в Google Оплата по КСГ: преимущества и недостатки
Оплата по КСГ: преимущества и недостатки презентация Царскосельский музей-лицей
презентация Царскосельский музей-лицей Христианин в труде. 4 класс
Христианин в труде. 4 класс Искусственный отбор. Породы собак
Искусственный отбор. Породы собак William Turner
William Turner Очистка трубопровода от отложений
Очистка трубопровода от отложений Острый респираторный дистресс-синдром при вирусных поражениях легких
Острый респираторный дистресс-синдром при вирусных поражениях легких Гражданская война в России
Гражданская война в России Рококо
Рококо Специальные звания органов внутренних дел
Специальные звания органов внутренних дел Слова с удвоенными согласными
Слова с удвоенными согласными Композиция в дизайне интерьера
Композиция в дизайне интерьера Пункт отбора на военную службу по контракту (2 разряда) г. Пемза
Пункт отбора на военную службу по контракту (2 разряда) г. Пемза Презентация Алкоголь и потомство
Презентация Алкоголь и потомство Шаблон оформления бизнес-плана
Шаблон оформления бизнес-плана Пероксисомы/Микротельца
Пероксисомы/Микротельца Биполярные транзисторы
Биполярные транзисторы Внутренние воды России. Реки. 8 класс
Внутренние воды России. Реки. 8 класс Реформы Петра I
Реформы Петра I Инструкция по заказу расчётно-справочной информации (справок, выписок) на сайте ПАО КБ Восточный
Инструкция по заказу расчётно-справочной информации (справок, выписок) на сайте ПАО КБ Восточный Қазақсандағы қазіргі діни жағдай
Қазақсандағы қазіргі діни жағдай